What Form 8655 Reduced 2024

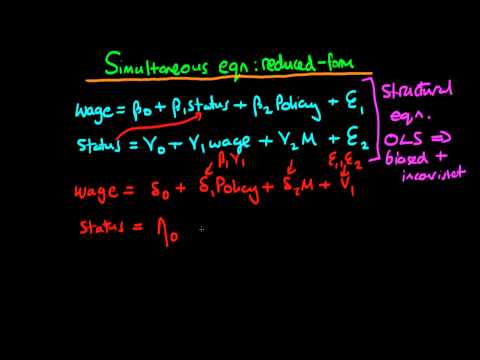
In this video, I want to talk about the reduced form and structural equations in simultaneous equation models. We're going to discuss the issue of both of these ways of writing down a symbolic model. Keeping with the example from last time, let's say an individual's wage is determined by their level of status in society. It is also dependent on some sort of governmental policy term, represented by the policy variable, as well as other factors within the error term epsilon 1. Additionally, we stipulate that status is dependent on an individual's level of wage and whether they are married, represented by the M term, as well as other factors within the error term epsilon 2. These two equations are referred to as the structural equations of our simultaneous equation model. They represent the underlying economics and our theory of what is happening. However, estimating these equations using ordinary least squares (OLS) can be biased and inconsistent due to the correlation between wage and epsilon 2 and the correlation between status and epsilon 1. In the last video, we derived another form of the equations. We found that we could write wage as a function of Delta naught, Delta 1 (the policy term), Delta 2 (whether an individual is married), and a composite error term B 1. To obtain this form, we substituted the second equation into the first equation. Delta 1 is a function of beta 1, gamma 1, and other coefficients, while V 1 is a function of epsilon 1 and epsilon 2. Similarly, we can find a corresponding equation for an individual's level of status. We can say that status is equal to eat a naught, e 2 1 (the policy variable), e 2 (whether an individual is married), and a composite error term B 2. These two equations...